Výzkumné centrum: Pokročilé sanační technologie a procesy

Mathematical description and theory of mixed-hydrid finite elements on the multidimensional (combined continuum and discrete fracture network) domain for the fluid flow problem – download paper

Mathematical description of the transport problem on the multidimensional domain (combined dual porosity continuum and discrete fracture network) – download paper

The basic idea of multidimensional model application results from the following facts:

• it is not possible to represent real structure of all fractures in larger scale, e.g. with the class of discrete fracture network (from both data availability and computational reasons)
• the equivalent continuum models are inappropriate simplification for detailed and coupled studies
• both large conductive fractures and small fractures are important either for the hydraulics or for the solute retention
• there is important role of large fracture intersections, behaving like ''pipe''

The combination of discrete and continuum models is now implemented in several finite element codes (e.g. FEFLOW, CONNECTFLOW) but there are still many limitations and open questions concerning, e.g. the generation of discretisation mesh for both 3D, 2D and 1D elements or the availability of appropriate input data.

Principle of the numerical discretisation of the multidimensional model – connection of 3D continuum and 2D and 1D discrete fracture network elements.

Difference between conforming (compatible) and non-conforming (incompatible) connection of continuum and fracture elements.

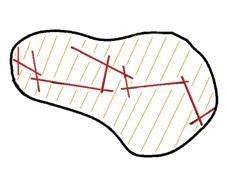Relation between real fracture network and multidimensional model: the hydraulically important fractures are represented explicitly while the small fractures are represented as continuum (possibly double-continuum for the dual-porosity transport model)

Scheme of the solution algorithm for the solute transport problem. We consider the multidimensional model and each part composed of mobile and immobile zone (dual-porosity continuum and dual-porosity fractures)

Ratio=500	50	5

Examples of solute transport in a combination of 2D continuum and 1D discrete fractures for variable ratio of fracture and continuum hydraulic conductivity.