Head of Laboratory: Dr. Radek Kolman
The Laboratory of Computational Solid Mechanics is engaged in analysis of nonlinear static and dynamic problems in the framework of finite element method in the fields of contact and impact of deformable bodies accompanied by geometric and material nonlinearities. Furthermore the wave propagation in heterogeneous and anisotropic environments by analytical and numerical methods and the evaluation and comparison of FEM models with experimental results are also fields of interest. In addition to this considerable effort is expended on the study of defects in crystalline materials experimentally and using the molecular dynamic method and on the electronic structure and total energy calculations of non-periodic systems using the finite element method or pseudopotential.
 PMD is a modern, platform-independent package of computer programs based on the Finite Element Method (FEM). The package is designed for general engineering problems in the continuum mechanics of solids. It is a proprietary code with a long, 25-year tradition, and is currently maintained by the staff of the Laboratory of Computational Solid Mechanics. The package also features its own GUI pre- and post-processor, GFEM.
PMD is a modern, platform-independent package of computer programs based on the Finite Element Method (FEM). The package is designed for general engineering problems in the continuum mechanics of solids. It is a proprietary code with a long, 25-year tradition, and is currently maintained by the staff of the Laboratory of Computational Solid Mechanics. The package also features its own GUI pre- and post-processor, GFEM.
Researchers: J. Plešek, J. Novotný, J. Dobiáš, S. Pták, D. Gabriel, P. Pařík, R. Kolman, Z. Hrubý, J. Kopačka, V. Sháněl, R. Marek
Collaboration: VAMET Ltd., www.vamet.cz
 Finite element modelling of wave propagation in solids and heterogenous media is proposed. The objective of the project lies in the improvement of current finite element models of transient dynamics problems related to wave propagation phenomena. The research concerns with comprehensive dispersion analysis of quadratic eight-node elements with the serendipity type shape functions, including accuracy, stability and optimization of different lumped matrices with variable mass distribution for such types of elements. In particular, the special attention is focused on the wave propagation in strongly heterogeneous media, homogenization techniques, high-velocity impact and sound waves in prestressed material.
Finite element modelling of wave propagation in solids and heterogenous media is proposed. The objective of the project lies in the improvement of current finite element models of transient dynamics problems related to wave propagation phenomena. The research concerns with comprehensive dispersion analysis of quadratic eight-node elements with the serendipity type shape functions, including accuracy, stability and optimization of different lumped matrices with variable mass distribution for such types of elements. In particular, the special attention is focused on the wave propagation in strongly heterogeneous media, homogenization techniques, high-velocity impact and sound waves in prestressed material.
Researchers: D. Gabriel, J. Plešek, R. Kolman, J. Červ, F. Valeš, J. Dobiáš
Collaboration: Faculty of Applied Sciences, University of West Bohemia in Pilsen
Related papers:
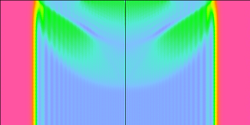 The project aims at the improvement of current computational modelling of contact-impact problems in the finite element method. The main objective lies in the development of original three dimensional algorithm based on the pre-discretization penalty method employing adequate numerical methods. As a part, new contact searching technique for the local search problems is proposed. The algorithm is implemented to the explicit solver of the FE code PMD (Package for Machine Design) for the solution of complex engineering problems.
The project aims at the improvement of current computational modelling of contact-impact problems in the finite element method. The main objective lies in the development of original three dimensional algorithm based on the pre-discretization penalty method employing adequate numerical methods. As a part, new contact searching technique for the local search problems is proposed. The algorithm is implemented to the explicit solver of the FE code PMD (Package for Machine Design) for the solution of complex engineering problems.
Researchers: D. Gabriel, J. Plešek, J. Kopačka, P. Pařík, R. Kolman, V. Sháněl, Z. Hrubý
Collaboration: Faculty of Mechanical Engineering, University of Maribor, Slovenia
Related papers:
 Success of new algorithms, suitable for efficient use on high-performance computers, substantially depends on properties of the mathematical methods they stem from. The objective of this project is development and application of such algorithms appropriate for solution to multiple non-linear problems of solid mechanics, while contact between solid deformable bodies, large displacements, finite rotations and material models with energy dissipation are considered as non-linear effects. Our approach is based on the FETI domain decomposition method and its variants. The numerical analysis of this method revealed that it is suitable for application to high-performance computers because it exhibits both parallel and numerical scalability. Contribution to theory of the FETI method, practically exploitable computer code implemented into the finite element package PMD and corresponding publications will be the outcome of the project.
Success of new algorithms, suitable for efficient use on high-performance computers, substantially depends on properties of the mathematical methods they stem from. The objective of this project is development and application of such algorithms appropriate for solution to multiple non-linear problems of solid mechanics, while contact between solid deformable bodies, large displacements, finite rotations and material models with energy dissipation are considered as non-linear effects. Our approach is based on the FETI domain decomposition method and its variants. The numerical analysis of this method revealed that it is suitable for application to high-performance computers because it exhibits both parallel and numerical scalability. Contribution to theory of the FETI method, practically exploitable computer code implemented into the finite element package PMD and corresponding publications will be the outcome of the project.
Researchers: J. Dobiáš, S. Pták, D. Gabriel
Collaboration: VŠB - Technical University of Ostrava
Related papers:
 A general knowledge about stability and growth conditions of fatigue cracks loaded under modes II, III and II+III is rather poor when compared to the mode I loading case. The project aims to apply new approaches to investigation of shear-mode cracks in order to better understand the crack-growth micromechanisms. Our part of the project is focused on an extended modelling of damage processes at the crack front via 3D molecular dynamics simulations. This technique enables us to obtain a multi-slip picture of dislocations in order to improve the classical dislocation models.
A general knowledge about stability and growth conditions of fatigue cracks loaded under modes II, III and II+III is rather poor when compared to the mode I loading case. The project aims to apply new approaches to investigation of shear-mode cracks in order to better understand the crack-growth micromechanisms. Our part of the project is focused on an extended modelling of damage processes at the crack front via 3D molecular dynamics simulations. This technique enables us to obtain a multi-slip picture of dislocations in order to improve the classical dislocation models.
Researchers: A. Machová, A. Uhnáková, P. Hora, V. Pelikán, O. Červená
Collaboration: Prof. J. Pokluda, Faculty of Mechanical Engineering, Brno University of Technology
Related papers:
 The aim of the project is to obtain new information on 3D acoustic emission sources coming from crack extension, twining, dislocation emission and crack front waves in 3D crystals of bcc iron and of other metals loaded in mode I by means of simulations on the atomistic level.
The aim of the project is to obtain new information on 3D acoustic emission sources coming from crack extension, twining, dislocation emission and crack front waves in 3D crystals of bcc iron and of other metals loaded in mode I by means of simulations on the atomistic level.
Researchers: J. Plešek, A. Machová, A. Uhnáková, P. Hora, V. Pelikán, O. Červená
Related papers:
 The spatial discretization of elastic continuum by Finite Element Method (FEM) introduces dispersion errors to numerical solutions of stress wave propagation. When these propagating phenomena are modeled by FEM the speed of a single harmonic wave depends on its frequency and thus a wave packet is distorted. Moreover, the oscillations near the sharp wavefront in FE solution (called Gibb’s effect) appears. For higher order Lagrangian finite elements (FEs) there are the optical modes in the spectrum resulting in spurious oscillations of stress and velocity distributions near the theoretical sharp wavefront. The main focus of dispersion analysis is devoted to higher order finite elements (mainly 20-node serendipity isoparametric finite element) and different modifications of shape functions (spectral FEM variant, hierarchical shape functions, splines, etc.). On the basis of dispersion analysis, the appropriate mesh size guarantees allowable dispersion errors and the accuracy of the numerical solution of dynamic response could be set.
The spatial discretization of elastic continuum by Finite Element Method (FEM) introduces dispersion errors to numerical solutions of stress wave propagation. When these propagating phenomena are modeled by FEM the speed of a single harmonic wave depends on its frequency and thus a wave packet is distorted. Moreover, the oscillations near the sharp wavefront in FE solution (called Gibb’s effect) appears. For higher order Lagrangian finite elements (FEs) there are the optical modes in the spectrum resulting in spurious oscillations of stress and velocity distributions near the theoretical sharp wavefront. The main focus of dispersion analysis is devoted to higher order finite elements (mainly 20-node serendipity isoparametric finite element) and different modifications of shape functions (spectral FEM variant, hierarchical shape functions, splines, etc.). On the basis of dispersion analysis, the appropriate mesh size guarantees allowable dispersion errors and the accuracy of the numerical solution of dynamic response could be set.
Researchers: R. Kolman, J. Plešek, M. Okrouhlík, D. Gabriel
Related papers:
 A modern approach in computational mechanics is the IsoGeometric Analysis (IGA). This numerical method employs shape functions based on different types of splines (B-splines, NURBS, T-splines and many others). The fields of unknown quantities are consequently described the same way as the geometry of the studied domain. In addition, this approach provides a higher degree of continuity than that offered by the classical finite element (FE) method based on Lagrangian polynomials. Isogeometric analysis aims to integrate FE ideas in CAD systems without necessity to regenerate mesh. The main focus of IGA research is its use for the numerical solution of the elastodynamics problems (free and forced vibration, propagating of elastic waves).
A modern approach in computational mechanics is the IsoGeometric Analysis (IGA). This numerical method employs shape functions based on different types of splines (B-splines, NURBS, T-splines and many others). The fields of unknown quantities are consequently described the same way as the geometry of the studied domain. In addition, this approach provides a higher degree of continuity than that offered by the classical finite element (FE) method based on Lagrangian polynomials. Isogeometric analysis aims to integrate FE ideas in CAD systems without necessity to regenerate mesh. The main focus of IGA research is its use for the numerical solution of the elastodynamics problems (free and forced vibration, propagating of elastic waves).
Researchers: R. Kolman, J. Plešek
Related papers:
 The project is focused on the development and application of numerical methods for the direct solution of large systems of linear equations, which are obtained by the finite element method (FEM) in continuum mechanics. A linear equation system is at the base of every FEM problem, therefore, it is necessary to be able to solve it as fast and efficient as possible. This is important especially in the nonlinear analysis, where the stiffness matrix has to be factorized repetitively. Large problems are usually problems whose requirements on the storage space and computational time make it difficult to obtain the solution using common computers. The work includes software implementation in PMD.
The project is focused on the development and application of numerical methods for the direct solution of large systems of linear equations, which are obtained by the finite element method (FEM) in continuum mechanics. A linear equation system is at the base of every FEM problem, therefore, it is necessary to be able to solve it as fast and efficient as possible. This is important especially in the nonlinear analysis, where the stiffness matrix has to be factorized repetitively. Large problems are usually problems whose requirements on the storage space and computational time make it difficult to obtain the solution using common computers. The work includes software implementation in PMD.
Researchers: P. Pařík, J. Plešek
Related papers:
 The goal of the project is the calibration and the numerical implementation of models involving so-called directional distortion hardening. The directional distortional hardening refers to the formation of a region of high curvature (sharpening) on the yield surface approximately in the direction of loading, and a region of low curvature (flattening) approximately in the opposite direction. It was experimentally observed that plastic deformations might cause the plastic anisotropy of materials that were isotropic initially.
The goal of the project is the calibration and the numerical implementation of models involving so-called directional distortion hardening. The directional distortional hardening refers to the formation of a region of high curvature (sharpening) on the yield surface approximately in the direction of loading, and a region of low curvature (flattening) approximately in the opposite direction. It was experimentally observed that plastic deformations might cause the plastic anisotropy of materials that were isotropic initially.
Researchers: J. Plešek, Z. Hrubý, R. Marek
Related papers:
 A particular attention is focused on the mass matrix diagonalization of the semiloof shell element. Its diagonalization requires a specially designed universal diagonalization scheme that is derived from the scaling HRZ method. Another analyzed aspect is the problem of preserving the moment of inertia for various types of finite elements. The proposed scheme is implemented in the finite element program PMD and consequently tested on several problems.
A particular attention is focused on the mass matrix diagonalization of the semiloof shell element. Its diagonalization requires a specially designed universal diagonalization scheme that is derived from the scaling HRZ method. Another analyzed aspect is the problem of preserving the moment of inertia for various types of finite elements. The proposed scheme is implemented in the finite element program PMD and consequently tested on several problems.
Researchers: V. Sháněl, R. Kolman, J. Plešek
Related papers:
© 2008–2012 Institute of Thermomechanics ASCR, v. v. i.