Diffractive optical elements
The operation of most passive optical elements is based on effects that occur at an interface between two homogeneous media. In traditional refractive optics the interface does not vary on the scale of the optical wavelength. Diffractive optical elements employ the controlled use of light propagation on microstructered interfaces. The recent emergence of microlithographic fabrication technology paved the way for fabricating optical devices with complicated structural features within the length-scale of optical waves. Such diffractive elements have many technological advantages and can be designed to perform functions unattainable with traditional optical devices. They provide the potential to significantly reduce the size, weight, and cost of a variety of optical systems. 
objective with diffractive lens (red) designed by Carl Zeiss AG
The practical application of diffractive optics technology has driven the need for mathematical models and numerical codes both to provide rigorous solutions of the Maxwell equations for complicated grating structures, thus predicting performance given the structure, and to carry out optimal design of new structures (cf. our program package WIAS-DiPoG). Moreover, the same numerical algorithms can be applied to the reconstruction of grating structures from their diffraction properties, i.e., to scatterometric measurements which will be described next.
Scatterometric measurement
The production of computer chips with increasingly small details requires a more and more accurate measurement technique. In particular, the development and evaluation of photolithographic manufacturing techniques necessitate a high precision measurement of special test geometries, which can be generated on the surface of lithographic masks and wafers. Besides well-established measurement techniques including AFM and SEM microscopy, the fast and non-destructive scatterometric methods are promising. In contrast to the optical microscopy, scatterometry does not produce a true image of the target. The geometry data is hidden in the measurement data and needs to be extracted by a mathematical optimization algorithm. In other words, an inverse problem for the reconstruction of the geometry data is to be solved.
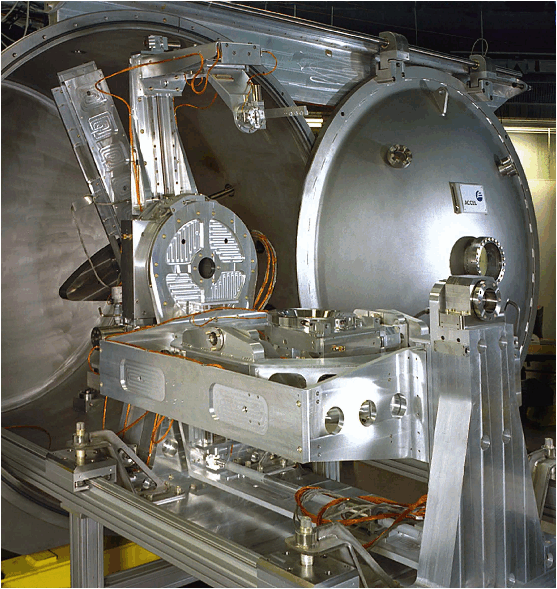
Measurement equipment at PTB/BESSY
The test geometries to be checked are simple periodic line-space structures (resp. biperiodic block structures). They are illuminated by light rays, and the distribution of the scattered wave is measured (cf. the measurement equipment of our cooperation partners at the PTB operating in the extreme UV range). Using the energy distribution and/or phase shifts of the scattered light, the geometry is to be reconstructed by mathematical optimization algorithms.
For real line-space structures, the desired rectangular cross section of the multi-layered lines (cf. Figure below) is changed to a trapezoidal shape, and the design values of the layer thicknesses are modified. Thus the measured side-wall angles and the deviations of thicknesses characterize the quality of the manufacturing process. In spite of working beyond the limits of diffraction (resp. in spite of the high degree of ill-posedness of the inverse problem), these geometry parameters can be determined with high precision. However, the impact of inaccurate material constants, the edge roundings, and stochastic perturbations of lines and interfaces are to be taken into account.
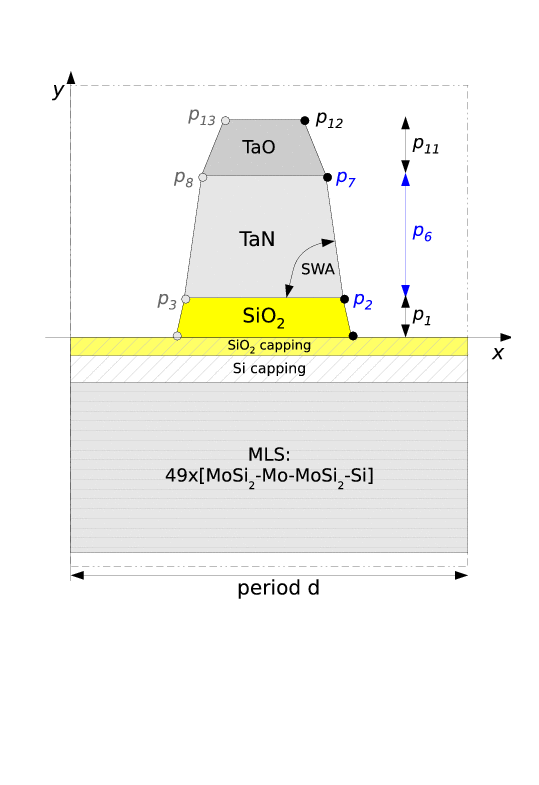
Cross section of one line in line-space grating
Publications
 Monographs
Monographs
-
D. Hömberg, G. Hu, eds., Issue on the workshop ``Electromagnetics -- Modelling, Simulation, Control and Industrial Applications'', 8, no. 3 of Discrete Contin. Dyn. Syst. Ser. S, American Institute of Mathematical Sciences, Springfield, 2015, 259 pages, (Collection Published).
-
L.I. Goray, G. Schmidt, Chapter 12: Boundary Integral Equation Methods for Conical Diffraction and Short Waves, in: Gratings: Theory and Numerical Applications, Second Revisited Edition, E. Popov, ed., Université d'Aix-Marseille, Institut Fresnel, Marseille, 2014, pp. 12.1--12.86, (Chapter Published).
-
P. Deuflhard, M. Grötschel, D. Hömberg, U. Horst, J. Kramer, V. Mehrmann, K. Polthier, F. Schmidt, Ch. Schütte, M. Skutella, J. Sprekels, eds., MATHEON -- Mathematics for Key Technologies, 1 of EMS Series in Industrial and Applied Mathematics, European Mathematical Society Publishing House, Zurich, 2014, 453 pages, (Collection Published).
 Articles in Refereed Journals
Articles in Refereed Journals
-
C. Brée, D. Gailevičius, V. Purlys, G.G. Werner, K. Staliunas, A. Rathsfeld, G. Schmidt, M. Radziunas, Chirped photonic crystal for spatially filtered optical feedback to a broad-area laser, Journal of Optics, 20 (2018), pp. 095804/1--095804/7, DOI 10.1088/2040-8986/aada98 .
Abstract
We derive and analyze an efficient model for reinjection of spatially filtered optical feedback from an external resonator to a broad area, edge emitting semiconductor laser diode. Spatial filtering is achieved by a chirped photonic crystal, with variable periodicity along the optical axis and negligible resonant backscattering. The optimal chirp is obtained from a genetic algorithm, which yields solutions that are robust against perturbations. Extensive numerical simulations of the composite system with our optoelectronic solver indicate that spatially filtered reinjection enhances lower-order transversal optical modes in the laser diode and, consequently, improves the spatial beam quality. -
T. Abbas, H. Ammari, G. Hu, A. Wahab, J.Ch. Ye, Elastic scattering coefficients and enhancement of nearly elastic cloaking, Journal of Elasticity, 128 (2017), pp. 203--243, DOI 10.1007/s10659-017-9624-7 .
Abstract
The concept of scattering coefficients has played a pivotal role in a broad range of inverse scattering and imaging problems in acoustic and electromagnetic media. In view of their promising applications, we introduce the notion of scattering coefficients of an elastic inclusion in this article. First, we define elastic scattering coefficients and substantiate that they naturally appear in the expansions of elastic scattered field and far field scattering amplitudes corresponding to a plane wave incidence. Then an algorithm is developed and analyzed for extracting the elastic scattering coefficients from multi-static response measurements of the scattered field. Moreover, the estimate of the maximal resolving order is provided in terms of the signal-to-noise ratio. The decay rate and symmetry of the elastic scattering coefficients are also discussed. Finally, we design scattering-coefficients-vanishing structures and elucidate their utility for enhancement of nearly elastic cloaking. -
G. Hu, A. Kirsch, T. Yin, Factorization method in inverse interaction problems with bi-periodic interfaces between acoustic and elastic waves, Inverse Problems and Imaging, 10 (2016), pp. 103--129.
Abstract
Consider a time-harmonic acoustic plane wave incident onto a doubly periodic (biperiodic) surface from above. The medium above the surface is supposed to be filled with homogeneous compressible inviscid fluid with a constant mass density, whereas the region below is occupied by an isotropic and linearly elastic solid body characterized by the Lamé constants. This paper is concerned with direct (or forward) and inverse fluid-solid interaction (FSI) problems with unbounded bi-periodic interfaces between acoustic and elastic waves. We present a variational approach to the forward interaction problem with Lipschitz interfaces. Existence of quasi-periodic solutions in Sobolev spaces is established at arbitrary frequency of incidence, while uniqueness is proved only for small frequencies or for all frequencies excluding a discrete set. Concerning the inverse problem, we show that the factorization method by Kirsch (1998) is applicable to the FSI problem in periodic structures. A computational criterion and a uniqueness result are justified for precisely characterizing the elastic body by utilizing the scattered acoustic near field measured in the fluid. -
Y. Guo, D. Hömberg, G. Hu, J. Li, H. Liu, A time domain sampling method for inverse acoustic scattering problems, Journal of Computational Physics, 314 (2016), pp. 647--660.
Abstract
This work concerns the inverse scattering problems of imaging unknown/inaccessible scatterers by transient acoustic near-field measurements. Based on the analysis of the migration method, we propose efficient and effective sampling schemes for imaging small and extended scatterers from knowledge of time-dependent scattered data due to incident impulsive point sources. Though the inverse scattering problems are known to be nonlinear and ill-posed, the proposed imaging algorithms are totally “direct” involving only integral calculations on the measurement surface. Theoretical justifications are presented and numerical experiments are conducted to demonstrate the effectiveness and robustness of our methods. In particular, the proposed static imaging functionals enhance the performance of the total focusing method (TFM) and the dynamic imaging functionals show analogous behavior to the time reversal inversion but without solving time-dependent wave equations. -
G. Hu, A. Rathsfeld, T. Yin, Finite element method to fluid-solid interaction problems with unbounded periodic interfaces, Numerical Methods for Partial Differential Equations. An International Journal, 32 (2016), pp. 5--35.
Abstract
Consider a time-harmonic acoustic plane wave incident onto a doubly periodic (biperiodic) surface from above. The medium above the surface is supposed to be filled with a homogeneous compressible inviscid fluid of constant mass density, whereas the region below is occupied by an isotropic and linearly elastic solid body characterized by its Lamé constants. This paper is concerned with a variational approach to the fluid-solid interaction problems with unbounded biperiodic Lipschitz interfaces between the domains of the acoustic and elastic waves. The existence of quasi-periodic solutions in Sobolev spaces is established at arbitrary frequency of incidence, while uniqueness is proved only for small frequencies or for all frequencies excluding a discrete set. A finite element scheme coupled with Dirichlet-to-Neumann mappings is proposed. The Dirichlet-to-Neumann mappings are approximated by truncated Rayleigh series expansions, and, finally, numerical tests in 2D are performed. -
G. Hu, H. Liu, Nearly cloaking the elastic wave fields, Journal de Mathématiques Pures et Appliquées, 104 (2015), pp. 1045--1074.
Abstract
In this work, we develop a general mathematical framework on regularized approximate cloaking of elastic waves governed by the Lamé system via the approach of transformation elastodynamics. Our study is rather comprehensive. We first provide a rigorous justification of the transformation elastodynamics. Based on the blow-up-a-point construction, elastic material tensors for a perfect cloak are derived and shown to possess singularities. In order to avoid the singular structure, we propose to regularize the blow-up-a-point construction to be the blow-up-a-small-region construction. However, it is shown that without incorporating a suitable lossy layer, the regularized construction would fail due to resonant inclusions. In order to defeat the failure of the lossless construction, a properly designed lossy layer is introduced into the regularized cloaking construction . We derive sharp asymptotic estimates in assessing the cloaking performance. The proposed cloaking scheme is capable of nearly cloaking an arbitrary content with a high accuracy. -
H. Gross, S. Heidenreich, M.-A. Henn, M. Bär, A. Rathsfeld, Modeling aspects to improve the solution of the inverse problem in scatterometry, Discrete and Continuous Dynamical Systems -- Series S, 8 (2015), pp. 497--519.
-
G. Hu, X. Liu, F. Qu, B. Zhang, Variational approach to rough surface scattering problems with Neumann and generalized impedance boundary conditions, Communications in Mathematical Sciences, 13 (2015), pp. 511--537.
-
G. Hu, A. Rathsfeld, Scattering of time-harmonic electromagnetic plane waves by perfectly conducting diffraction gratings, IMA Journal of Applied Mathematics, 80 (2015), pp. 508--532.
Abstract
Consider scattering of time-harmonic electromagnetic plane waves by a doubly periodic surface in $R^3$. The medium above the surface is supposed to be homogeneous and isotropic with a constant dielectric coefficient, while below is a perfectly conducting material. This paper is concerned with the existence of quasiperiodic solutions for any frequency of incidence. Based on an equivalent variational formulation established by the mortar technique of Nitsche, we verify the existence of solutions for a broad class of incident waves including plane waves, under the assumption that the grating profile is a Lipschitz biperiodic surface. Our solvability result covers the resonance case where a Rayleigh frequency is allowed. Non-uniqueness examples are also presented in the resonance case and the TE or TM polarization case for classical gratings. -
TH. Arnold, A. Rathsfeld, Reflection of plane waves by rough surfaces in the sense of Born approximation, Mathematical Methods in the Applied Sciences, 37 (2014), pp. 2091--2111.
Abstract
The topic of the present paper is the reflection of electromagnetic plane waves by rough surfaces, i.e., by smooth and bounded perturbations of planar faces. Moreover, the contrast between the cover material and the substrate beneath the rough surface is supposed to be low. In this case, a modification of Stearns' formula based on Born approximation and Fourier techniques is derived for a special class of surfaces. This class contains the graphs of functions if the interface function is a radially modulated almost periodic function. For the Born formula to converge, a sufficient and almost necessary condition is given. A further technical condition is defined, which guarantees the existence of the corresponding far field of the Born approximation. This far field contains plane waves, far-field terms like those for bounded scatterers, and, additionally, a new type of terms. The derived formulas can be used for the fast numerical computations of far fields and for the statistics of random rough surfaces. -
G. Hu, A. Rathsfeld, Convergence analysis of the FEM coupled with Fourier-mode expansion for the electromagnetic scattering by biperiodic structures, Electronic Transactions on Numerical Analysis, 41 (2014), pp. 350--375.
-
G. Schmidt, Conical diffraction by multilayer gratings: A recursive integral equations approach, Applications of Mathematics, 58 (2013), pp. 279--307.
Abstract
In this paper we consider an integral equation algorithm to study the scattering of plane waves by multilayer diffraction gratings under oblique incidence. The scattering problem is described by a system of Helmholtz equations with piecewise constant coefficients in $R^2$ coupled by special transmission conditions at the interfaces between different layers. Boundary integral methods lead to a system of singular integral equations, containing at least two equations for each interface. To deal with an arbitrary number of material layers we present the extension of a recursive procedure developed by Maystre for normal incidence, which transforms the problem to a sequence of equations with $2 times 2$ operator matrices on each interface. Necessary and sufficient conditions for the applicability of the algorithm are derived. -
G. Hu, F. Qu, B. Zhang, A linear sampling method for inverse problems of diffraction gratings of mixed type, Mathematical Methods in the Applied Sciences, 35 (2012), pp. 1047--1066.
-
G. Hu, Inverse wave scattering by unbounded obstacles: Uniqueness for the two-dimensional Helmholtz equation, Applicable Analysis. An International Journal, 91 (2012), pp. 703--717.
Abstract
In this paper we present some uniqueness results on inverse wave scattering by unbounded obstacles for the two-dimensional Helmholtz equation. We prove that an impenetrable one-dimensional rough surface can be uniquely determined by the values of the scattered field taken on a line segment above the surface that correspond to the incident waves generated by a countable number of point sources. For penetrable rough layers in a piecewise constant medium, the refractive indices together with the rough interfaces (on which the TM transmission conditions are imposed) can be uniquely identified using the same measurements and the same incident point source waves. Moreover, a Dirichlet polygonal rough surface can be uniquely determined by a single incident point source wave provided a certain condition is imposed on it. -
L. Goray, G. Schmidt, Analysis of two-dimensional photonic band gaps of any rod shape and conductivity using a conical-integral-equation method, Physical Review E. Statistical, Nonlinear, and Soft Matter Physics, 85 (2012), pp. 036701/1--036701/12.
Abstract
The conical boundary integral equation method has been proposed to calculate the sensitive optical response of 2D photonic band gaps (PBGs), including dielectric, absorbing, and high-conductive rods of various shapes working in any wavelength range. It is possible to determine the diffracted field by computing the scattering matrices separately for any grating boundary profile. The computation of the matrices is based on the solution of a 2 x 2 system of singular integral equations at each interface between two different materials. The advantage of our integral formulation is that the discretization of the integral equations system and the factorization of the discrete matrices, which takes the major computing time, are carried out only once for a boundary. It turned out that a small number of collocation points per boundary combined with a high convergence rate can provide adequate description of the dependence on diffracted energy of very different PBGs illuminated at arbitrary incident and polarization angles. The numerical results presented describe the significant impact of rod shape on diffraction in PBGs supporting polariton-plasmon excitation, particularly in the vicinity of resonances and at high filling ratios. The diffracted energy response calculated vs. array cell geometry parameters was found to vary from a few percent up to a few hundred percent. The influence of other types of anomalies (i.e. waveguide anomalies, cavity modes, Fabry-Perot and Bragg resonances, Rayleigh orders, etc), conductivity, and polarization states on the optical response has been demonstrated. -
H. Gross, M.-A. Henn, S. Heidenreich, A. Rathsfeld, M. Bär, Modeling of line roughness and its impact on the diffraction intensities and the reconstructed critical dimensions in scatterometry, Applied Optics, 51 (2012), pp. 7384--7394.
Abstract
We investigate the impact of line edge and line width roughness (LER, LWR) on the measured diffraction intensities in angular resolved extreme ultraviolet (EUV) scatterometry for a periodic line-space structure designed for EUV lithography. LER and LWR with typical amplitudes of a few nanometers were previously neglected in the course of the profile reconstruction. The 2D rigorous numerical simulations of the diffraction process for periodic structures are carried out with the finite element method (FEM) providing a numerical solution of the two-dimensional Helmholtz equation. To model roughness, multiple calculations are performed for domains with large periods, containing many pairs of line and space with stochastically chosen line and space widths. A systematic decrease of the mean efficiencies for higher diffraction orders along with increasing variances is observed and established for different degrees of roughness. In particular, we obtain simple analytical expressions for the bias in the mean efficiencies and the additional uncertainty contribution stemming from the presence of LER and/or LWR. As a consequence this bias can easily be included into the reconstruction model to provide accurate values for the evaluated profile parameters. We resolve the sensitivity of the reconstruction from this bias by using the LER/LWR perturbed efficiency datasets for multiple reconstructions. If the scattering efficiencies are bias-corrected, significant improvements are found in the reconstructed bottom and top widths toward the nominal values. -
M.-A. Henn, S. Heidenreich, H. Gross, A. Rathsfeld, F. Scholze, M. Bär, Improved grating reconstruction by determination of line roughness in extreme ultraviolet scatterometry, Optics Letters, 37 (2012), pp. 5229--5231.
-
C. Pfüller, M. Ramsteiner, O. Brandt, F. Grosse, A. Rathsfeld, G. Schmidt, L. Geelhaar, H. Riechert, Raman spectroscopy as a probe for the coupling of light into ensembles of sub-wavelength-sized nanowires, Applied Physics Letters, 101 (2012), pp. 083104/1--083104/4.
-
G. Hu, B. Zhang, The linear sampling method for the inverse electromagnetic scattering by a partially coated bi-periodic structure, Mathematical Methods in the Applied Sciences, 34 (2011), pp. 509--519.
-
F. Lanzara, V. Maz'ya, G. Schmidt, On the fast computation of high dimensional volume potentials, Mathematics of Computation, 80 (2011), pp. 887--904.
-
F. Lanzara, V.G. Maz'ya, G. Schmidt, Accurate cubature of volume potentials over high-dimensional half-spaces, Journal of Mathematical Sciences (New York), 173 (2011), pp. 683--700.
-
J. Elschner, G. Hu, Inverse scattering of elastic waves by periodic structures: Uniqueness under the third or fourth kind boundary conditions, Methods and Applications of Analysis, 18 (2011), pp. 215--244.
Abstract
The inverse scattering of a time-harmonic elastic wave by a two-dimensional periodic structure in $R^2$ is investigated. The grating profile is assumed to be a graph given by a piecewise linear function on which the third or fourth kind boundary conditions are satisfied. Via an equivalent variational formulation, existence of quasi-periodic solutions for general Lipschitz grating profiles is proved by applying the Fredholm alternative. However, uniqueness of solution to the direct problem does not hold in general. For the inverse problem, we determine and classify all the unidentifiable grating profiles corresponding to a given incident elastic field, relying on the reflection principle for the Navier equation and the rotational invariance of propagating directions of the total field. Moreover, global uniqueness for the inverse problem is established with a minimal number of incident pressure or shear waves, including the resonance case where a Rayleigh frequency is allowed. The gratings that are unidentifiable by one incident elastic wave provide non-uniqueness examples for appropriately chosen wave number and incident angles. -
J. Elschner, G. Hu, Uniqueness in inverse scattering of elastic waves by three-dimensional polyhedral diffraction gratings, Journal of Inverse and Ill-Posed Problems, 19 (2011), pp. 717--768.
Abstract
We consider the inverse elastic scattering problem of determining a three-dimensional diffraction grating profile from scattered waves measured above the structure. In general, a grating profile cannot be uniquely determined by a single incoming plane wave. We completely characterize and classify the bi-periodic polyhedral structures under the boundary conditions of the third and fourth kinds that cannot be uniquely recovered by only one incident plane wave. Thus we have global uniqueness for a polyhedral grating profile by one incident elastic plane wave if and only if the profile belongs to neither of the unidentifiable classes, which can be explicitly described depending on the incident field and the type of boundary conditions. Our approach is based on the reflection principle for the Navier equation and the reflectional and rotational invariance of the total field. -
J. Elschner, G. Hu, Uniqueness in inverse transmission scattering problems for multilayered obstacles, Inverse Problems and Imaging, 5 (2011), pp. 793--813.
Abstract
Assume a time-harmonic electromagnetic wave is scattered by an infinitely long cylindrical conductor surrounded by an unknown piecewise homogenous medium remaining invariant along the cylinder axis. We prove that, in TM mode, the far field patterns for all observation directions at a fixed frequency uniquely determine the unknown surrounding medium as well as the shape of the cylindrical conductor. A similar uniqueness result is obtained for the scattering by multilayered penetrable periodic structures in a piecewise homogenous medium. The periodic interfaces and refractive indices can be uniquely identified from the near field data measured only above (or below) the structure for all quasi-periodic incident waves with a fixed phase-shift. The proofs are based on the singularity of the Green function to a two dimensional elliptic equation with piecewise constant leading coefficients. -
G. Schmidt, B.H. Kleemann, Integral equation methods from grating theory to photonics: An overview and new approaches for conical diffraction, Journal of Modern Optics, 58 (2011), pp. 407--423.
-
G. Schmidt, Integral equations for conical diffraction by coated gratings, Journal of Integral Equations and Applications, 23 (2011), pp. 71--112.
Abstract
The paper is devoted to integral formulations for the scattering of plane waves by diffraction gratings under oblique incidence. For the case of coated gratings Maxwell's equations can be reduced to a system of four singular integral equations on the piecewise smooth interfaces between different materials. We study analytic properties of the integral operators for periodic diffraction problems and obtain existence and uniqueness results for solutions of the systems corresponding to electromagnetic fields with locally finite energy. -
G. Hu, F. Qu, B. Zhang, Direct and inverse problems for electromagnetic scattering by a doubly periodic structure with a partially coated dielectric, Mathematical Methods in the Applied Sciences, 33 (2010), pp. 147--156.
-
S. Chandler-Wilde, J. Elschner, Variational approach in weighted Sobolev spaces to scattering by unbounded rough surface, SIAM Journal on Mathematical Analysis, 42 (2010), pp. 2554--2580.
-
L.I. Goray, G. Schmidt, Solving conical diffraction grating problems with integral equations, Journal of the Optical Society of America A. Optics, Image Science, and Vision, 27 (2010), pp. 585--597.
Abstract
Off-plane scattering of time-harmonic plane waves by a diffraction grating with arbitrary conductivity and general border profile is considered in a rigorous electromagnetic formulation. The integral equations for conical diffraction were obtained using the boundary integrals of the single and double layer potentials including the tangential derivative of single layer potentials interpreted as singular integrals. We derive an important formula for the calculation of the absorption in conical diffraction. Some rules which are expedient for the numerical implementation of the theory are presented. The efficiencies and polarization angles compared with those obtained by Lifeng Li for transmission and reflection gratings are in a good agreement. The code developed and tested is found to be accurate and efficient for solving off-plane diffraction problems including high-conductive surfaces, borders with edges, real border profiles, and gratings working at short wavelengths. -
H. Gross, J. Richter, A. Rathsfeld, M. Bär, Investigations on a robust profile model for the reconstruction of 2D periodic absorber lines in scatterometry, Journal of the European Optical Society - Rapid Publications, 5 (2010), pp. 10053/1--10053/7.
-
J. Elschner, G. Hu, Global uniqueness in determining polygonal periodic structures with a minimal number of incident plane waves, Inverse Problems. An International Journal on the Theory and Practice of Inverse Problems, Inverse Methods and Computerized Inversion of Data, 26 (2010), pp. 115002/1--115002/23.
Abstract
In this paper, we investigate the inverse problem of recovering a two-dimensional perfectly reflecting diffraction grating from the scattered waves measured above the structure. Inspired by a novel idea developed by Bao, Zhang and Zou [to appear in Trans. Amer. Math. Soc.], we present a complete characterization of the global uniqueness in determining polygonal periodic structures using a minimal number of incident plane waves. The idea in this paper combines the reflection principle for the Helmholtz equation and the dihedral group theory. We characterize all periodic polygonal structures that cannot be identified by one incident plane wave, including the resonance case where a Rayleigh frequency is allowed. Furthermore, we show that those unidentifiable gratings provide non-uniqueness examples for appropriately chosen wave number and incident angles. We also indicate and fix a gap in the proof of the main theorem of Elschner and Yamamoto [Z. Anal. Anwend., 26 (2007), 165-177], and generalize the uniqueness results of that paper. -
H. Gross, A. Rathsfeld, F. Scholze, M. Bär, Profile reconstruction in extreme ultraviolet (EUV) scatterometry: Modeling and uncertainty estimates, Measurement Science and Technology, 20 (2009), pp. 105102/1--105102/11.
Abstract
Scatterometry as a non-imaging indirect optical method in wafer metrology is also relevant to lithography masks designed for Extreme Ultraviolet Lithography, where light with wavelengths in the range of 13 nm is applied. The solution of the inverse problem, i.e. the determination of periodic surface structures regarding critical dimensions (CD) and other profile properties from light diffraction patterns, is incomplete without knowledge of the uncertainties associated with the reconstructed parameters. With decreasing feature sizes of lithography masks, increasing demands on metrology techniques and their uncertainties arise. The numerical simulation of the diffraction process for periodic 2D structures can be realized by the finite element solution of the two-dimensional Helmholtz equation. For typical EUV masks the ratio period over wave length is so large, that a generalized finite element method has to be used to ensure reliable results with reasonable computational costs. The inverse problem can be formulated as a non-linear operator equation in Euclidean spaces. The operator maps the sought mask parameters to the efficiencies of diffracted plane wave modes. We employ a Gauß-Newton type iterative method to solve this operator equation and end up minimizing the deviation of the measured efficiency or phase shift values from the calculated ones. We apply our reconstruction algorithm for the measurement of a typical EUV mask composed of TaN absorber lines of about 80 nm height, a period of 420 nm resp. 720 nm, and with an underlying MoSi-multilayer stack of 300 nm thickness. Clearly, the uncertainties of the reconstructed geometric parameters essentially depend on the uncertainties of the input data and can be estimated by various methods. We apply a Monte Carlo procedure and an approximative covariance method to evaluate the reconstruction algorithm. Finally, we analyze the influence of uncertainties in the widths of the multilayer stack by the Monte Carlo method. -
H. Gross, A. Rathsfeld, Sensitivity analysis for indirect measurement in scatterometry and the reconstruction of periodic grating structures, Waves in Random and Complex Media. Propagation, Scattering and Imaging, 18 (2008), pp. 129--149.
-
J. Elschner, M. Yamamoto, Uniqueness in determining polygonal periodic structures, Zeitschrift fur Analysis und ihre Anwendungen. Journal for Analysis and its Applications, 26 (2007), pp. 165--177.
-
H. Gross, R. Model, M. Bär, M. Wurm, B. Bodermann, A. Rathsfeld, Mathematical modelling of indirect measurements in scatterometry, Measurement, 39 (2006), pp. 782--794.
-
A. Rathsfeld, G. Schmidt, B.H. Kleemann, On a fast integral equation method for diffraction gratings, Communications in Computational Physics, 1 (2006), pp. 984-1009.
-
G. Bruckner, J. Elschner, The numerical solution of an inverse periodic transmission problem, Mathematical Methods in the Applied Sciences, 28 (2005), pp. 757--778.
-
J. Elschner, M. Yamamoto, Uniqueness results for an inverse periodic transmission problem, Inverse Problems. An International Journal on the Theory and Practice of Inverse Problems, Inverse Methods and Computerized Inversion of Data, 20 (2004), pp. 1841--1852.
-
G. Bao, K. Huang, G. Schmidt, Optimal design of nonlinear diffraction gratings, Journal of Computational Physics, 184 (2003), pp. 106--121.
-
G. Bruckner, J. Elschner, A two-step algorithm for the reconstruction of perfectly reflecting periodic profiles, Inverse Problems. An International Journal on the Theory and Practice of Inverse Problems, Inverse Methods and Computerized Inversion of Data, 19 (2003), pp. 315--329.
-
J. Elschner, G.C. Hsiao, A. Rathsfeld, Grating profile reconstruction based on finite elements and optimization techniques, SIAM Journal on Applied Mathematics, 64 (2003), pp. 525--545.
-
J. Elschner, G. Schmidt, M. Yamamoto, An inverse problem in periodic diffractive optics: Global uniqueness with a single wavenumber, Inverse Problems. An International Journal on the Theory and Practice of Inverse Problems, Inverse Methods and Computerized Inversion of Data, 19 (2003), pp. 779--787.
-
J. Elschner, G. Schmidt, M. Yamamoto, Global uniqueness in determining rectangular periodic structures by scattering data with a single wave number, Journal of Inverse and Ill-Posed Problems, 11 (2003), pp. 235--244.
-
J. Elschner, G. Schmidt, Conical diffraction by periodic structures: Variation of interfaces and gradient formulas, Mathematische Nachrichten, 252 (2003), pp. 24--42.
 Contributions to Collected Editions
Contributions to Collected Editions
-
C. Brée, V. Raab, D. Gailevičius, V. Purlys, J. Montiel, G.G. Werner, K. Staliunas, A. Rathsfeld, U. Bandelow, M. Radziunas, Genetically optimized photonic crystal for spatial filtering of reinjection into broad-area diode lasers, in: 2019 Conference on Lasers and Electro-Optics Europe and European Quantum Electronics Conference, OSA Technical Digest, IEEE, Piscataway, 2019, pp. 1--1, DOI 10.1109/CLEO-EQEC.2019.8871622 .
Abstract
Modern high-power broad-area semiconductor laser diodes (BASLDs) deliver optical output powers of several ten Watts at high electro-optical conversion efficiencies, which makes them highly relevant for numerous industrial, medical and scientific applications. However, lateral multimode behavior in BASLDs due to thermal lensing turns out highly detrimental, as it results in poor focusability and decreased laser beam brightnesss. Approaches to overcome this issue include improved epitaxial layer design, the optimization of evanescent spatial filtering by tailoring the emitter geometry and facet reflectivity, or Fourier spatially filtered reinjection from an external resonator [1]. -
G. Schmidt, Boundary integral methods for periodic scattering problems, in: Around the Research of Vladimir Maz'ya II. Partial Differential Equations, A. Laptev, ed., 12 of International Mathematical Series, Springer Science+Business Media, New York [et al.], 2010, pp. 337--363.
-
H. Gross, F. Scholze, A. Rathsfeld, M. Bär, Evaluation of measurement uncertainties in EUV scatterometry, in: Modeling Aspects in Optical Metrology II, H. Bosse, B. Bodermann, R.M. Silver, eds., 7390 of Proceedings of SPIE, SPIE, 2009, pp. 7390OT/1--7390OT/11.
-
H. Gross, A. Rathsfeld, M. Bär, Modelling and uncertainty estimates for numerically reconstructed profiles in scatterometry, in: Advanced Mathematical and Computational Tools in Metrology and Testing VIII, F. Pavese, M. Bär, A.B. Forbes, J.M. Linares, C. Perruchet, N.F. Zhang, eds., 78 of Series on Advances in Mathematics for Applied Sciences, World Scientific, Singapore, 2009, pp. 142--147.
-
M.-A. Henn, R. Model, M. Bär, M. Wurm, B. Bodermann, A. Rathsfeld, H. Gross, On numerical reconstructions of lithographic masks in DUV scatterometry, in: Modeling Aspects in Optical Metrology II, H. Bosse, B. Bodermann, R.M. Silver, eds., 7390 of Proceedings of SPIE, SPIE, 2009, pp. 7390OQ/1--7390OQ/11.
-
H. Gross, R. Model, A. Rathsfeld, F. Scholze, M. Wurm, B. Bodermann, M. Bär, Modellbildung, Bestimmung der Messunsicherheit und Validierung für diskrete inverse Probleme am Beispiel der Scatterometrie, in: Sensoren und Messsysteme, 14. Fachtagung Ludwigsburg, 11./12. März 2008, 2011 of VDI-Berichte, VDI, 2008, pp. 337--346.
-
R. Model, A. Rathsfeld, H. Gross, M. Wurm, B. Bodermann, A scatterometry inverse problem in optical mask metrology, in: 6th International Conference on Inverse Problems in Engineering: Theory and Practice, 15--19 June 2008, Dourdan (Paris), France, 135 of J. Phys.: Conf. Ser., Inst. Phys., 2008, pp. 012071/1--012071/8.
-
H. Gross, A. Rathsfeld, F. Scholze, M. Bär, U. Dersch, Optimal sets of measurement data for profile reconstruction in scatterometry, in: Modeling Aspects in Optical Metrology, H. Bosse, B. Bodermann, R.M. Silver, eds., 6617 of Proceedings of SPIE, 2007, pp. 66171B/1--66171B/12.
-
M. Wurm, B. Bodermann, F. Scholze, Ch. Laubis, H. Gross, A. Rathsfeld, Untersuchung zur Eignung der EUV-Scatterometrie zur quantitativen Charakterisierung periodischer Strukturen auf Photolithographiemasken, in: Proc. of the 107th Meeting of DGaO (German Branch of the European Optical Society), June 6--10, 2006, in Weingarten, DGaO-Proceedings, 2006, pp. P74/1--P74/2.
-
P. DE Bisschop, A. Erdmann, A. Rathsfeld, Simulation of the effect of a resist-surface bound air bubble on imaging in immersion lithography, in: Optical Microlithography XVIII, B.W. Smith, ed., 5754 of Proceedings of SPIE, 2005, pp. 243--253.
-
G. Bruckner, J. Elschner, M. Yamamoto, An optimization method for the grating profile reconstruction, Proceedings 3rd ISAAC Congress, Berlin, August 20 - 25, 2001, H.G.W. Begehr, R.P. Gilbert, M.W. Wong, eds., II of Progress in Analysis, World Scientific, New Jersey [u.a.], 2003, pp. 1391--1404.
-
J. Elschner, R. Hinder, G. Schmidt, Direct and inverse problems for diffractive structures --- Optimization of binary gratings, in: Mathematics --- Key Technology for the Future. Joint Projects Between Universities and Industry, W. Jäger, H.-J. Krebs, eds., Springer, Berlin [u.a.], 2003, pp. 293--304.
-
G. Schmidt, Electromagnetic scattering by periodic structures (in Russian), Proceedings of the International Conference on Differential and Functional Differential Equations, Moscow, Russian Federation, August 11 - 17, 2002, 3 of Sovrem. Probl. Mat. Fund. Naprav., 2003, pp. 113--128.
 Preprints, Reports, Technical Reports
Preprints, Reports, Technical Reports
-
G. Hu, A. Rathsfeld, Radiation conditions for the Helmholtz equation in a half plane filled by inhomogeneous periodic material, Preprint no. 2726, WIAS, Berlin, 2020, DOI 10.20347/WIAS.PREPRINT.2726 .
Abstract, PDF (439 kByte)
In this paper we consider time-harmonic acoustic wave propagation in a half-plane filled by inhomogeneous periodic medium. If the refractive index depends on the horizontal coordinate only, we define upward and downward radiating modes by solving a one-dimensional Sturm-Liouville eigenvalue problem with a complex-valued periodic coefficient. The upward and downward radiation conditions are introduced based on a generalized Rayleigh series. Using the variational method, we then prove uniqueness and existence for the scattering of an incoming wave mode by a grating located between an upper and lower half plane with such inhomogeneous periodic media. Finally, we discuss the application of the new radiation conditions to the scattering matrix algorithm, i.e., to rigorous coupled wave analysis or Fourier modal method. -
A. Rathsfeld, On a half-space radiation condition, Preprint no. 2669, WIAS, Berlin, 2019, DOI 10.20347/WIAS.PREPRINT.2669 .
Abstract, PDF (341 kByte)
For the Dirichlet problem of the Helmholtz equation over the half space or rough surfaces, a radiation condition is needed to guarantee a unique solution, which is physically meaningful. If the Dirichlet data is a general bounded continuous function, then the well-established Sommerfeld radiation condition, the angular spectrum representation, and the upward propagating radiation condition do not apply or require restrictions on the data, in order to define the involved integrals. In this paper a new condition based on a representation of the second derivative of the solution is proposed. The twice differentiable half-space Green's function is integrable and the corresponding radiation condition applies to general bounded functions. The condition is checked for special functions like plane waves and point source solution. Moreover, the Dirichlet problem for the half plane is discussed. Note that such a “continuous” radiation condition is helpful e.g. if finite sections of the rough-surface problem are analyzed. -
G. Hu, A. Rathsfeld, Acoustic scattering from locally perturbed periodic surfaces, Preprint no. 2522, WIAS, Berlin, 2018, DOI 10.20347/WIAS.PREPRINT.2522 .
Abstract, PDF (298 kByte)
We prove well-posedness for the time-harmonic acoustic scattering of plane waves from locally perturbed periodic surfaces in two dimensions under homogeneous Dirichlet boundary conditions. This covers sound-soft acoustic as well as perfectly conducting, TE polarized electromagnetic boundary value problems. Our arguments are based on a variational method in a truncated bounded domain coupled with a boundary integral representation. If the quasi-periodic Green's function to the unperturbed periodic scattering problem is calculated efficiently, then the variational approach can be used for a numerical scheme based on coupling finite elements with a boundary element algorithm.
Even for a general 2D rough-surface problem, it turns out that the Green's function defined with the radiation condition ASR satisfies the Sommerfeld radiation condition over the half plane. Based on this result, for a local perturbation of a periodic surface, the scattered wave of an incoming plane wave is the sum of the scattered wave for the unperturbed periodic surface plus an additional scattered wave satisfying Sommerfeld's condition on the half plane. Whereas the scattered wave for the unperturbed periodic surface has a far field consisting of a finite number of propagating plane waves, the additional field contributes to the far field by a far-field pattern defined in the half-plane directions similarly to the pattern known for bounded obstacles.
 Talks, Poster
Talks, Poster
-
C. Brée, V. Raab, D. Gailevičius, V. Purlys, J. Montiel, G.G. Werner, K. Staliunas, A. Rathsfeld, U. Bandelow, M. Radziunas, Genetically optimized photonic crystal for spatial filtering of reinjection into broad-area diode lasers, CLEO/Europe-EQEC 2019, Munich, June 23 - 27, 2019.
-
G. Hu, Direct and inverse problems in elastodynamics, Workshop ``Theory, Numerics and Application of Partial Differential Equations'', December 10, 2016, Chinese Academy of Sciences, Beijing, December 10, 2016.
-
G. Hu, Inverse medium scattering problems, Workshop on Inverse Problems and their Applications, November 18 - 20, 2016, Southeast University, Nanjing, China, November 20, 2016.
-
D.R.M. Renger, Functions of bounded variation with an infinite-dimensional codomain, Meeting in Applied Mathematics and Calculus of Variations, September 13 - 16, 2016, Università di Roma ``La Sapienza'', Dipartimento di Matematica ``Guido Castelnuovo'', Italy, September 16, 2016.
-
G. Schmidt, Scattering of general incident beams by diffraction gratings, European Optical Society Annual Meeting (EOSAM) 2016, September 26 - 30, 2016, Berlin, September 28, 2016.
-
G. Hu, Mathematical problems in time-harmonic wave scattering, Shandong Normal University, Department of Mathematics, Jinan, China, November 11, 2014.
-
G. Hu, Mathematical problems in time-harmonic wave scattering from bounded and unbounded obstacles, South University of Science and Technology of China (SUSTC), Department of Financial Mathematics and Financial Engineering, Shenzhen, November 3, 2014.
-
J. Elschner, Direct and inverse problems for diffraction gratings, Symposium ``Light Scattering: Simulation and Inversion'', May 27 - 28, 2013, Bremen, May 28, 2013.
-
G. Schmidt, On an integral equation formulation for scattering by biperiodic structures, Analysis of Partial Differential Equations, A Symposium in honour of Prof. Vladimir Maz'ya on the occasion of his 75th birthday, December 16 - 17, 2013, University of Liverpool, UK, December 17, 2013.
-
G. Hu, Direct and inverse scattering of elastic waves by diffraction gratings, 6th International Conference ``Inverse Problems, Control and Shape Optimization'' (PICOF '12), April 2 - 4, 2012, Palaiseau, France, April 4, 2012.
-
G. Hu, Direct and inverse scattering of elastic waves by diffraction gratings, Workshop 3 ``Wave Propagation and Scattering, Inverse Problems and Applications in Energy and the Environment'', November 21 - 25, 2011, Johann Radon Institute for Computational and Applied Mathematics (RICAM), Linz, Austria, November 24, 2011.
-
N. Kleemann, Shape derivatives for conical diffraction by non-smooth interfaces, Technische Universität Berlin, Institut für Mathematik, January 6, 2011.
-
N. Kleemann, Shape derivatives for conical diffraction by non-smooth interfaces, Friedrich-Schiller-Universität Jena, Mathematisches Institut, February 11, 2011.
-
A. Rathsfeld, On Born approximation for the scattering by rough surfaces, 262. PTB Seminar, EUV Metrology, October 27 - 28, 2011, Physikalisch-Technische Bundesanstalt, Berlin, October 28, 2011.
-
G. Schmidt, Fast computation of high-dimensional volume potentials, Trilateral Workshop on Separation of Variables and Applications (SVA), September 8 - 10, 2010, La Colle-sur-Loup, France, September 10, 2010.
-
G. Schmidt, Integral methods for conical diffraction by multi-profile gratings, Annual International Conference ``Days on Diffraction 2010'', June 8 - 11, 2010, St. Petersburg, Russian Federation, June 9, 2010.
-
G. Schmidt, On the computation of volume potentials over high-dimensional rectangular domains, The First Workshop on Approximate Approximations and Their Applications, December 14 - 15, 2010, University of Liverpool, UK, December 15, 2010.
-
A. Rathsfeld, Modelling and algorithms for simulation and reconstruction in scatterometry, Workshop on Scatterometry and Ellipsometry on Structured Surfaces, March 18 - 19, 2009, Physikalisch-Technische Bundesanstalt, Department ``Imaging and Wave Optics'', Braunschweig, March 18, 2009.
-
A. Rathsfeld, Numerical aspects of the scatterometric measurement of periodic surface structures, Conference on Applied Inverse Problems 2009, July 20 - 24, 2009, University of Vienna, Austria, July 21, 2009.
-
G. Schmidt, Existence and uniqueness of solution for a system of Helmholtz equations, International Conference on Elliptic and Parabolic Equations, November 30 - December 4, 2009, WIAS, December 3, 2009.
-
A. Rathsfeld, Scatterometry: Inverse problems and optimization of measurements, University of Tokyo, Department of Mathematical Sciences, Japan, March 6, 2008.
-
G. Schmidt, Integral equations for conical diffraction by coated gratings, Annual International Conference ``Days on Diffraction'', June 3 - 6, 2008, St. Petersburg, Russian Federation, June 3, 2008.
-
J. Elschner, On uniqueness in inverse scattering by obstacles and diffraction gratings, Conference ``Boundary Elements --- Theory and Applications'' (Beta 2007), May 22 - 24, 2007, Leibniz Universität Hannover, May 22, 2007.
-
J. Elschner, On uniqueness in inverse scattering with finitely many incident waves, Workshop ``Inverse Problems in Wave Scattering'', March 5 - 9, 2007, Mathematisches Forschungsinstitut Oberwolfach, March 6, 2007.
-
A. Rathsfeld, Sensitivity analysis for indirect measurement in scatterometry and the reconstruction of periodic grating structures, 6th International Congress on Industrial and Applied Mathematics (ICIAM 2007), July 16 - 20, 2007, ETH Zürich, Switzerland, July 20, 2007.
-
J. Elschner, Inverse problems for diffraction gratings, Waves Meeting, September 21 - 23, 2006, University of Reading, UK, September 22, 2006.
-
J. Elschner, Variational approach to scattering by unbounded surfaces, 12th Conference on Mathematics of Finite Elements and Applications (MAFELAP 2006), June 13 - 16, 2006, Brunel University, Uxbridge, UK, June 15, 2006.
-
J. Elschner, Variational approach to scattering by unbounded surfaces, Autumn School ``Analysis of Maxwell's Equations'' (Research Training Group GRK 1294 ``Analysis, Simulation and Design of Nanotechnological Processes''), October 17 - 19, 2006, Universität Karlsruhe, October 18, 2006.
-
A. Rathsfeld, Inverses Problem, Sensitivitätsanalyse, optimierte Messstrategie, BMBF-Projekttreffen ABBILD, Physikalisch-Technische Bundesanstalt, Berlin, November 13, 2006.
-
A. Rathsfeld, Sensitivity analysis for scatterometry and reconstruction of periodic grating structures, Physikalisch-Technische Bundesanstalt, Berlin, October 26, 2006.
-
J. Elschner, Inverse Probleme für optische Gitter, Physikalisch-Technische Bundesanstalt, Berlin, April 13, 2005.
-
J. Elschner, Inverse problems for diffraction gratings, Inverse Scattering Workshop, University of North Carolina, Charlotte, USA, June 3, 2005.
-
J. Elschner, Inverse problems for diffraction gratings, University of Delaware, Department of Mathematics, Newark, USA, June 9, 2005.
-
A. Rathsfeld, Finite elements for the rigorous simulation of time-harmonic waves, 3rd IISB Lithography Simulation Workshop, September 16 - 18, 2005, Pommersfelden, September 16, 2005.
-
A. Rathsfeld, Integralgleichungsmethode für optische Gitter --- Weiterentwicklung der IESMP, Kick-off Meeting of BMBF Project ``NAOMI'', Carl Zeiss AG, Jena, May 31, 2005.
-
A. Rathsfeld, Local optimization of polygonal gratings for classical and conical diffraction, Conference ``Diffractive Optics 2005'', Warsaw, Poland, September 3 - 7, 2005.
-
A. Rathsfeld, Local optimization of polygonal gratings for classical and conical diffraction, WIAS Workshop ``New Trends in Simulation and Control of PDEs'', September 26 - 28, 2005, Berlin, September 26, 2005.
-
A. Rathsfeld, Optimierung von optischen Gittern mit tt DiPoG-2.1, 2nd Meeting ``Inverses Problem in der Scatterometrie'', Physikalisch-Technische Bundesanstalt, Braunschweig, October 18, 2005.
-
A. Rathsfeld, Optimization of diffraction gratings with tt DiPoG, sc Matheon MF 1 Workshop ``Optimization Software'', Konrad-Zuse-Zentrum für Informationstechnik Berlin, June 1, 2005.
-
G. Schmidt, Simulation und Optimierung periodischer diffraktiver Strukturen mit DiPoG, Physikalisch-Technische Bundesanstalt, Berlin, April 13, 2005.
-
J. Elschner, Direct and inverse problems for the periodic Helmholtz equation I, University of Tokyo, Department of Mathematical Sciences, Japan, February 12, 2004.
-
J. Elschner, Direct and inverse problems for the periodic Helmholtz equation II, University of Tokyo, Department of Mathematical Sciences, Japan, February 13, 2004.
-
J. Elschner, Inverse scattering for diffraction gratings, European Congress on Computational Methods in Applied Sciences and Engineering (ECCOMAS 2004), June 24 - 28, 2004, Jyväskylä, Finland, June 26, 2004.
-
J. Elschner, Inverse scattering of plane waves from periodic surfaces, Chemnitzer Minisymposium 2004 zu Inversen Problemen, Universität Chemnitz, September 23, 2004.
-
J. Elschner, Recent progress in inverse periodic diffraction problems, Workshop ``Mathematical Analyses and Numerical Methods for Applied Inverse Problems'', January 19 - 21, 2004, University of Tokyo, Japan, January 20, 2004.
-
A. Rathsfeld, Simulation und Optimierung diffraktiver Strukturen für die Mikrooptik, Seminar des Forschungsschwerpunktes Photonik, Technische Universität Berlin, Optisches Institut, October 22, 2004.
-
G. Bruckner, J. Elschner, A. Rathsfeld, G. Schmidt, Simulation, optimization and reconstruction of diffractive structures, Conference ``Diffractive Optics 2003'', Oxford, UK, September 17 - 20, 2003.
-
J. Elschner, Inverse problems for diffraction gratings: Uniqueness results, Meeting ``Inverse Problems in Wave Scattering and Impedance Tomography'', April 20 - 25, 2003, Mathematisches Forschungsinstitut Oberwolfach, April 22, 2003.
-
J. Elschner, Inverse problems for periodic diffractive structures, Meeting ``Functional Analysis and Partial Differential Equations'', June 2 - 3, 2003, Han-sur-Lesse, Belgium, June 3, 2003.
-
J. Elschner, On the numerical solution of inverse periodic transmission problems, University of Tokyo, Department of Mathematical Sciences, Japan, August 5, 2003.
 External Preprints
External Preprints
-
G. Hu, B. Zhang, The linear sampling method for the inverse electromagnetic scattering by a partially coated bi-periodic structure, Preprint no. arXiv:1003.3067, Cornell University Library, arXiv.org, 2010.
-
J. Elschner, G. Schmidt, M. Yamamoto, An inverse problem in periodic diffractive optics: Global uniqueness with a single wave number, Preprint no. 5, University of Tokyo, Graduate School of Mathematical Sciences, 2003.


